Why turning a ket into a cat may or may not be a good idea#
People often ask me what I do. There is a lot of things I can talk about: why is a quantum computer interesting or useful , or what do I actually do during my day. But quite often people end up asking a confused question about this curious story of an undead cat. In this blog post I will try to shed some light on this case as well as delve into the question of why we use these kind of stories.

Dead? Or alive? Or both?#
When trying to understand a new theory we physicists love our thought experiments. We take some mathematical model of the world, change some parameters to see how it behaves and try to extract some rules of thumb or intuition from it.
An example that most people will be familiar with is that of gravity. In its simplest form Newton’s law of gravitation just says that objects with mass attract each other. However if we play around a bit with a toy model we can learn that small and light objects tend to orbit large and heavy objects such as the sun.
An advantage of thought experiments over real experiments is that, besides being much easier to conduct/execute, it is possible to push a model to its extreme. It gets interesting when the model predicts things that go against our (preexisting) physical intuition. This is the point where either the model breaks down, or where we predict a new phenomenon. An example would be what happens when an object becomes so heavy that the gravitational attraction between its constituent particles becomes so large that it collapses in on itself.
One of the virtues of having a mathematical description of a system is that you can make an analogy explicit. This allows one to use intuition from one system to better understand some other system. An excellent example is the analogue between an LC-circuit and a kid on the swing. Both of these systems are described by the mathematics of the simple harmonic oscillator. Because both have the same mathematical description we can explain how driving an electrical circuit behaves in the same way as pushing a kid on a swing. The power of such an analogy is evident as most people have far more intuition as to what it means to push a kid on a swing than what it means to resonantly drive an LC circuit.
When trying to explain a physical phenomenon we often use similar analogies to show where a person’s preexisting intuition applies to the problem at hand, and where it does not. The idea of using an analogy to explain something is often simplified to the advice: “talk about something people can relate to”. A problem occurs when something relatable such as a cat is used but the rules that apply have nothing to do with that similarity, resulting in confusion instead of clarity.
A relatable zombie cat#
I think that one of the worst ways to explain quantum mechanics is using Schrödinger’s cat. In this experiment, a cat is placed in a box with a vial of poison, some radioactive material, a hammer and a Geiger counter. If the radioactive material decays this will be detected by the Geiger counter and the hammer will smash the vial, resulting in a lethal dose of poison for our furry friend. Now quantum mechanics says that as long as we do not open the box, the radioactive particle will be in a superposition of both having and not having decayed. Similarly, the cat, which is entangled with the state of the radioactive particle, is in a superposition of being both dead and alive at the same time. Wait, what ?!?
The reason why I think that Schrödinger’s cat is a terrible way of explaining quantum mechanics is that all it does is state that according to quantum mechanics the cat is both dead and alive at the same time. Not only does this go against your intuition, it provides no explanation other than “quantum says so”, while at the same time introducing terms like superposition and entanglement. All it achieves is a feeling that quantum mechanics is some kind of magic that nobody truly understands.
It is not surprising that Schrödinger’s cat is such a confusing example. Schrödinger explicitly devised the paradox to highlight problems with the Copenhagen interpretation of Quantum Mechanics, which would state that the cat is indeed both dead and alive at the same time.
Waves or particles ?#
There are, however, better ways of introducing quantum mechanics. My personal favorite is the double slit experiment, an absolutely beautiful experiment made famous by Richard Feynman Richard Feynamn . The double slit experiment was originally used to resolve a dispute dating back to Newton and Huygens about the nature of light. Newton held that light consisted of tiny particles while Huygens proposed a theory describing light as waves.
In order to resolve this question, we need to devise an experiment that shows light behaving in a way that can only be explained if light consists of either waves or particles. If you need some intuition for the characteristic behavior of waves, imagine that you have a small pond of water. If you throw a stone in the pond, you’ll see waves spreading outwards in a circle. If you throw two stones in the pond just apart from each other you will see two such circles moving outward, where the two waves hit each other their amplitudes get added up, producing a characteristic pattern, this is called interference. In the double slit experiment we are going to look for interference as a characteristic of wave-like behavior.

Water exhibiting wave like behavior.#
The double slit experiment (Figure below) consists of a light source (S) that shoots light towards a screen (a) with two holes, hole 1 and hole 2. Behind the screen, there is a backstop where we detect the intensity of the light on each position x. Let’s start with the simple case where we block one of the two holes, say hole 2. Now if we are sending particles at hole 1, we would expect most of the light to end up directly behind the hole, with some spread because the particles may hit the edges of the hole. Similarly if we are sending waves through the hole, we would expect the hole to act like a wave source with the light spreading out from it. This would result in the largest intensity directly behind the hole. Both of these cases would result in a distribution of the light intensity that looks like $P_1$, independent of the wave or particle nature of light.
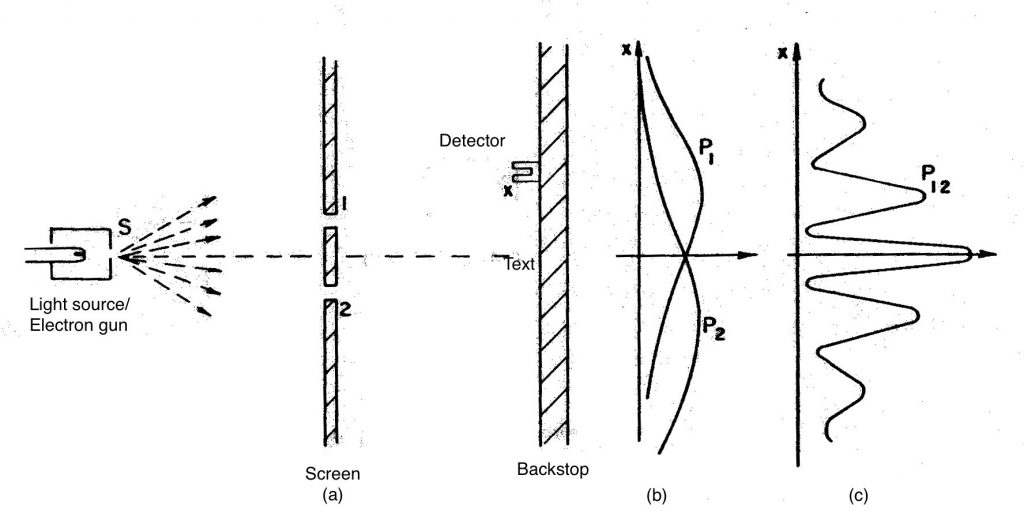
Figure adapted from The Feynman Lectures Vol III Fig 3.1.#
It gets more interesting when we open up both holes. In the case of particles we would expect each particle to go either through hole 1 or hole 2, resulting in a distribution that looks like the sum of $P_1$ and $P_2$. If on the other hand, light consists of waves we would expect to be able to see interference, similar to the pattern we get when throwing stones in the water, resulting in a distribution that looks like $P_{12}$. In 1803 Thomas Young performed exactly this experiment, observing interference and clearly deciding the case in favour of the wave theory of light.
Wavy particles#
If this was all there was to it I wouldn’t be telling this story. It so happens that in the late 19th century physics was facing another crisis, known as the ultraviolet catastrophe. I will not go into the details here but for the sake of this discussion it is enough to know that theories based on the continuous wave nature of light predicted that a hot object (blackbody) would emit infinite amounts of energy, something that is clearly at odds with observation! The eventual resolution of this problem came when Planck proposed that light can only be emitted in discrete packages. Einstein later postulated that these “light quanta” correspond to real particles, now known as photons [1] .
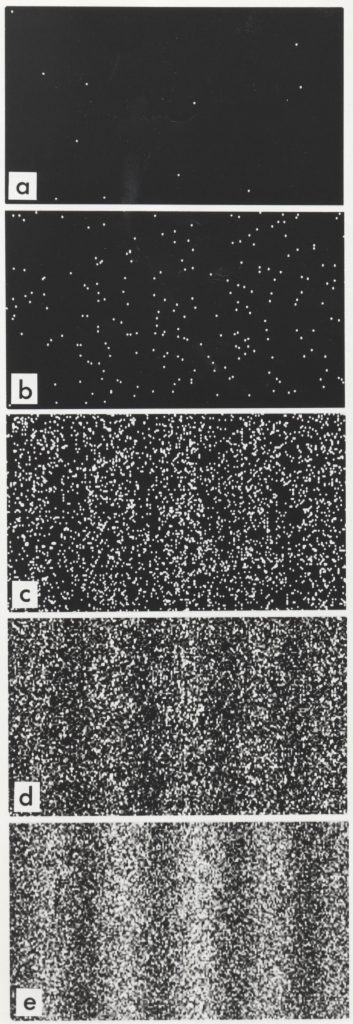
Electron buildup over time for a double slit experiment using electrons.#
Now if you get a bit suspicious here you are right, did I not just tell you that light is a wave and not a particle? Ok, maybe light consists of particles but their motion is described by waves. In fact, quantum mechanics describes particles using a wave equation known as the Schrodinger equation.
Only there is a bit of a problem here. If we repeat the double slit experiment described above with single particles, we would not expect to see interference. If we make our light source so weak that only a single particle is send through the setup each time, then there should be nothing for this particle to interfere with. However when such an experiment is performed, the interference does not disappear. Instead, each particle that goes through the apparatus lands on a seemingly random location with probabilities described by distribution $P_{12}$. The figure below shows exactly this effect using electrons, but using photons would give the same result.
The problem gets even weirder when we try to answer the question of which hole the particle went through. Suppose that we measure what hole the particle goes through. The moment we measure the particle going through one of the two holes, the particle acts exactly as if the other hole is closed and the interference disappears completely. The very act of measuring disturbs the system and forces the particle to make a choice as to what hole to go through!
The reason I like the double slit experiment so much is that it takes some phenomena that most people are familiar with and combines them into an experiment that highlights some of the most fundamental characteristics of quantum mechanics. It does this by taking preexisting intuition on how (water) waves behave and exploring the consequences of adding the simple premise that these waves come in discrete quanta.
Footnotes
Note
This post originally appeared on bits of quantum.
Comments
Comments by giscus, use a GitHub account to comment.